
|
|

|
||
 Appendix A: Design Procedure for Riprap Armor
models can be used to determine depth-averaged velocities but are rarely justified
due to cost. Figure A.2 presents an empirical method to estimate the ratio
by R/W and aspect ratio. The following notation is used:
Vss
= characteristic side slope velocity (maximum at any point along bend)
(length/time),
Vavg
= average channel velocity at upstream end of bend in the main channel
only (length/time),
R
= center-line radius of bend (length), and
W
= water-surface width of the main channel, length (note that W here
should not be confused with stone weight).
Velocity downstream of bends decays at approximately the following rate: No
decay in first channel width of bend exit; decay of Vss/Vavg = 0.1 per channel
width until Vss/Vavg = 1.0. For straight channels sufficiently far (>5W-10W)
from upstream bends, Vss/Vavg shown in Figure A.3 are recommended.
However, few channels are straight enough to use Vss/Vavg < 1. See Figure A.4
for a description of VSS and Figure A.5 for the location in a trapezoidal channel
bend where the maximum near-bank velocity was located. Figure A.6 shows the
variation in velocity over the side slope in the exit region downstream of a bend.
Figures A.4, A.5, and A.6 are presented to illustrate concepts; the designer
should consider the specific geometry. For equal cross-sectional areas, steep side
slopes tend to move the maximum bend velocities away from the side slope;
whereas, mild side slopes allow the maximum bend velocities to occur over the
side slope. Analytical methods are velocity estimation, such as velocities
resulting from subsections of a water-surface profile computation, should be used
only in straight reaches, in which case the velocity from the subsection adjacent
to the bank subsection should be used as VSS in design of bank riprap. Appendix
G in EM 1110-2-1601 provides a velocity estimation method based on using
observed field data to estimate riprap design velocities.
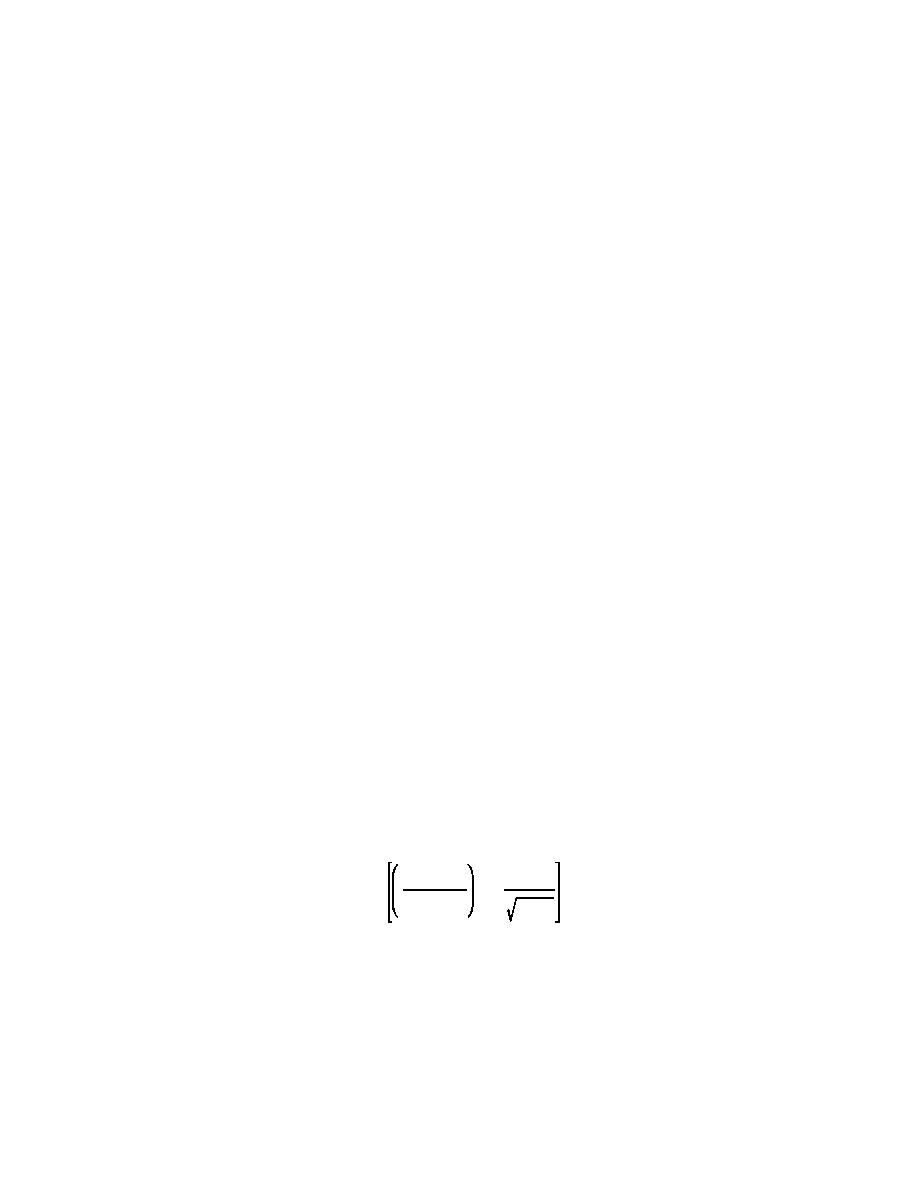
(2) Stone Size Relations. The basic equation for the representative stone size in
straight or curved channels is
2.5
1/2
W
V
D30 ' SfCsCvCTd
(A.3)
s & W
K1gd
A-12
|
||
 |
||