If we are designing a verification study, pilot studies, such as the one described in examples 1
and 2, provide a priori knowledge of D and SD. This information can be used to design the
verification study with a sample size large enough to ensure that the power is as great as
desired. This is accomplished through increasing the sample size until the desired value for β is
achieved on the operating characteristic curve.
Example 1: Columbia River Camas/Washougal Station--Hand Calculation
The following example illustrates this method with data from the Camas/Washougal total
dissolved gas monitoring station (CWMW) on the Columbia River. To assist smolt in their
downstream migration, the U.S. Army Corps of Engineers spills surface water from projects on
the Columbia and Snake Rivers. This spillage causes air to be driven into the water column to
depths where it causes gases in the water column to be supersaturated with respect to surface
saturation. This supersaturation can be detrimental to fish, so the Corps monitors spill gas
concentrations in the rivers. Thus, this system is designed to determine the extreme total
dissolved gas concentrations resulting from spilling water. This information is used for
compliance and in project operations.
To determine if these monitors could be used to determine the flux of total dissolved gas in
the river, the statistical verification studies presented in this technical note were carried out. The
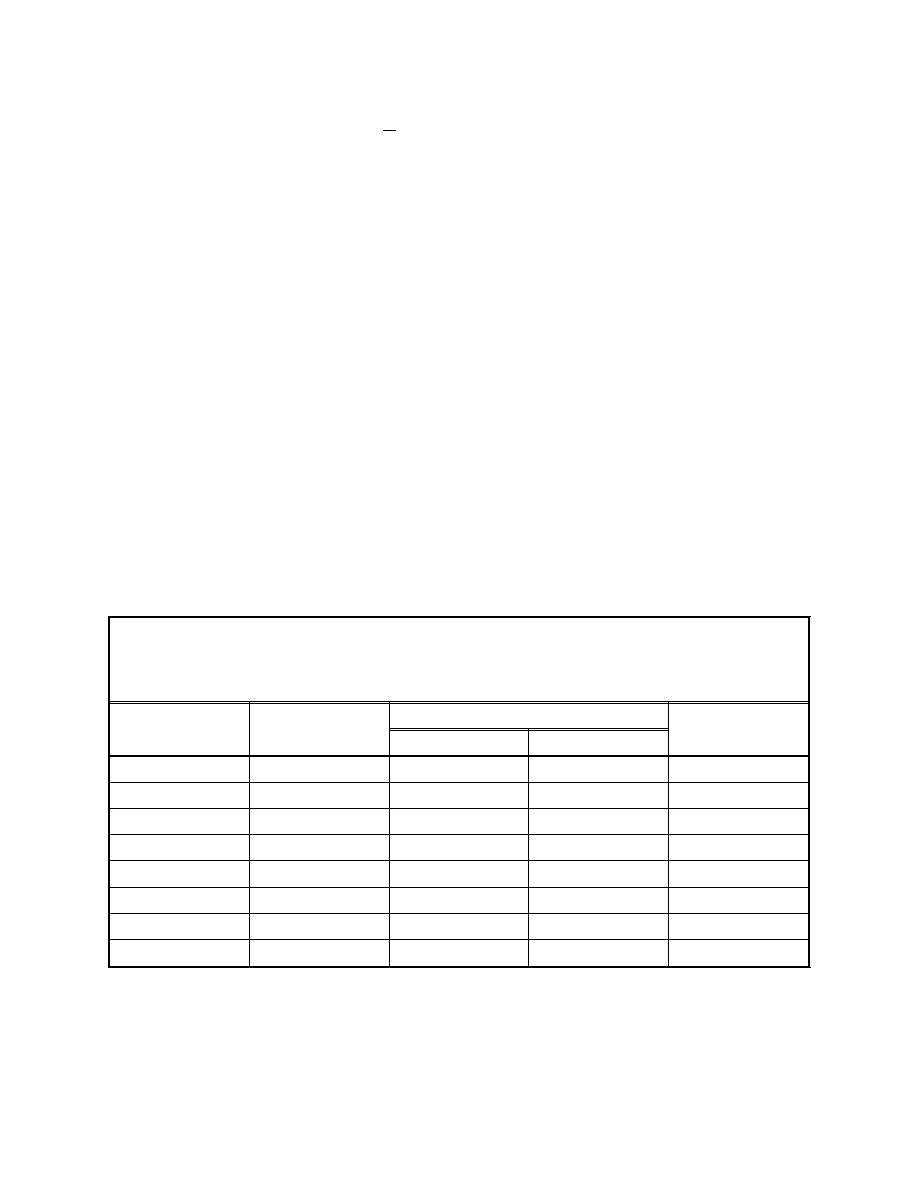
verification is based on comparing monitor data with data collected at eight transects near the
CWMW monitor site (river mile 122) on 3 days (Table 1). The stations on the transects were
approximately evenly spaced, so the data for each transect were simply averaged together to
obtain an average total dissolved gas concentration at that transect.
Table 1
Average Total Dissolved Gas as Percent Saturation, Columbia River Transects and
Camas/Washougal Monitoring Station Fixed Monitor
Percent Saturation
Date
Transect Mile
Transect Average
Monitor
No. Samples
18 May 95
119.9
115.1
113.4
5
25 May 95
121.2
118.1
115.5
5
25 May 95
121.6
119.0
117.3
5
25 May 95
122.1
119.4
118.5
5
25 May 95
119.9
117.0
113.4
7
27 Jul 95
121.2
112.1
109.8
32
27 Jul 95
121.6
116.0
111.9
15
27 Jul 95
122.1
112.9
109.5
15
Figures 4 and 5 show normal probability plots of the transect and fixed monitor system data,
respectively. Ideally, the data would be randomly distributed along the normal distribution line,
with points close to and on either side of the line. Though the transect data in Figure 4 do not
appear to be completely random about the normal line, they are sufficiently normal for this
6
Water Quality Technical Note AM-03 (January 1998)


 Previous Page
Previous Page
